probability attitude
I was thinking just now in the bath tub if I could summarize the lessons learned from this huge success of the last two weeks.
The causes of this huge success were, first of all, my new probability thinking/attitude, and second of all, luck.
That's right, because the very lesson I learned, by adopting probability thinking, is the first reason that makes me state that I was "lucky", as it always is. Good luck or bad luck. Even if your chances are 99% of making it, if you don't fail because of that 1%, you could say you were "lucky" (not according to the dictionary meaning, of course). You were lucky because the event was probable but not certain.
And the other lesson I learned is to appraise correctly the probability of that good luck coming your way.
The change in attitude was brought about by the little probability theory I studied at Khan Academy and on this awesome little website, apparently for children:
Probability
The big thing I learned from reading probability theory and doing those little games/exercises is when I applied it to my trading and to the concept of "drawdown" and "maximum drawdown".
Drawdown and probability are very close, closer than what dictionaries and online glossaries lead you to think. In particular the general understanding of "maximum drawdown" is very faulty.
The existence of the concept itself of "maximum drawdown" is a sign of the problem, because "maximum drawdown" does not mean very much and is not very useful. What is useful is to assess correctly the probability of different levels of drawdown.
You see, if trading systems (and portfolios of trading systems) are like flipping coins, and they are for the purpose of our discussion (even though with different odds), then saying "maximum drawdown" of a system is like saying "maximum consecutive heads of a coin", and we know that does not mean anything, because the possible consecutive heads are infinite, and the only issue is
how probable they are rather than if they are
possible. In the same way "maximum drawdown" doesn't mean anything, and yet everyone is talking about it.
Drawdown Definition | Wikinvest
Reduction in account equity from a trade or series of trades.
Drawdown Definition | Investopedia
The peak-to-trough decline during a specific record period of an investment, fund or commodity.
Drawdown (economics) - Wikipedia, the free encyclopedia
The Drawdown is the measure of the decline from a historical peak in some variable.
Well, actually, so far, everything would seem ok. As far as the definition, I have nothing to argue, but the problem is all the assumptions that people attach to that concept, and particularly to the concept of "maximum drawdown" (even though often "drawdown" is used as a synonym for "maximum drawdown"). The problem is when people like this guy, and almost everyone, including myself until recently, write and think stuff like this (red part is bad, green part is good):
Wikinvest News - Forex Systems: What Is Drawdown?
[...]
Why Is It Useful?
Having a figure for the drawdown of a system is a very useful measure of the risk that you are likely to encounter. Clearly it depends on how much risk you are placing on each trade, so knowing this figure will help you keep your per trade risk within boundaries where your funds are not likely to be wiped out when things go against you. It gives you a worst case scenario based on past results.
If your actual loss goes to more than you have on deposit with your broker or the credit you have agreed with him, your broker will ask for more money (issue a margin call) or close your position. Having a drawdown figure for your system helps you to avoid this by making sure that your expected potential loss is covered.
Some caution is required here because you cannot assume that the past results are necessarily going to continue into the future. You should plan for a buffer, probably at least as much again.
[...]
He's first wrong, when he says "worst case scenario", then right, when he says "caution", then wrong again, when he says "at least as much again".
Now, given that - for simplification purposes - trades from systems are like tossing coins (cfr.
this post), would you ever say "worst case scenario" for consecutive heads? There you go. And of course "caution" is the right word, but then again, no amount of money will make you secure from the risk of blowing out your account, so saying "probably at least as much again" is at best misleading. And yet this is what everyone has been thinking, including myself until recently. But there is no need to further discuss this: I have clarified how the concept of "maximum drawdown" as a guarantee of safety is an illusion. It's not a matter of what amount of capital makes you safe from drawdown, but of how the probability of blowing out decreases as your capital increases.
So you cannot have a capital that will make you safe from "maximum drawdown" because you cannot estimate the "maximum drawdown", which potentially is infinite. What you do know, with systems' trades as with coins is that if you toss a coin, your chance of having two heads in a row 1/2 times 1/2 equal 1/4 (25%), and so on. And that your chance of having two losses in a row with two systems (or one system producing two trades) that have 66% win rate and 33% losing rate, is 0.33 times 0.33 equal 11%.
The fact that in the past your consecutive losses are 5 at the most does not mean you won't exceed them, but it only means your past is not long enough to give you a correct assessment of the probability. So, also consecutive losses are meaningless. Percentage of wins matters, and so does size of wins.
For example, if you have 5 maximum consecutive losses with systems that have a 66% win rate, your chance of getting them is 0.33^5 = 0.4%, so having a sample of 200 trades would lead you to think that the biggest number of consecutive losses is 5. But with a sample of 1000 trades, you'd probably find out that you can also have 6 consecutive losses, since their probability is 0.33^6 = 0.14%, which means one and a half every 1000 trades. And then, with a sample of 10,000 trades, you'd find out that there is also a chance of getting 7 consecutive losses, and that is 0.33^7 = 0.05%, which means one instance every two thousands trades. So would you still regard as holy the concept of "maximum consecutive losses"? You'd probably stop using it altogether.
So, if maximum drawdown (and maximum consecutive losses) basically doesn't mean or guarantee anything, and we're only up against the
probability of failure rather than the
possibility of failure, then things change, a lot.
Because, once understood this much, I realized that I no longer had to wait to gather a capital that could make me "safe" from the maximum drawdown of my systems. There was no such capital. So it was only a matter of appraising the probability of failure by resuming trading with whatever capital I had (4000).
And here's what I did. First of all, resampling. I took all the backtested relativized trades of the selected portfolio, multiplied them by 10, mixed them up (by assigning a random number to each trade), and came up with a resampled data that excluded lucky combinations (one system winning after another one losing), so I assumed no (bad) correlation of all the back-tested relativized trades, but also no (good) inverse correlation, because the back-tested sample showed that my systems were somewhat inversely correlated (some losing when others winning), so I figured I couldn't assume this to be the typical scenario, I wanted to rule out what could have been a lucky combination, and preferred to base my estimates on a random sampling of the same back-tested trades. And, once again, i mixed them up randomly to avoid the risk of portfolio curve-fitting. I just repeat myself all the time, to be clear.
Then I calculated the maximum drawdowns (plural) by starting on every single day of the 65,000 days I had. Yes, once again, I am using the concept that I said was meaningless. But even
then, I stressed out that... "the frequency of past losses tells us the frequency of future losses, and the frequency of strings of losses (drawdown) tells us the future likelihood of that, too". I talked about "likelihood", which means probability.
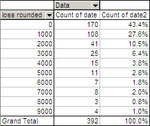
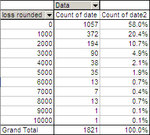
So, after 1) relativization, 2) resampling, 3) calculating the dradowns, I came up with this table:
I produced several other samples with 65,000 trades, and each time I got very similar values.
From the table, I figured that by starting on any given day, I had a 77% of losing 2000 dollars or less, and therefore that same chance of not blowing out my 4000 dollars account (by losing more than 2000, because at that point you can't trade anymore).
This information, even though we know reality is worse, because systems underperform in the future, tells me much more than the "maximum" drawdown, which is a laughable concept. But even that "18,000" at the end of the table doesn't mean much. It only means that, out of 65,000 days you could start trading, there's 11 days where you will blow out your account unless you have 20 thousand dollars account. But there will clearly be a chance in a million days that we will exceed that 18,000, and lose 30,000. And there will be a chance in 10 millions that we will lose 40,000 (always assuming, for simplification purposes, throughout this post, that the systems do not underperform the past, or even stop being profitable).
So, that table, too, has limits, and it's not safe, inasmuch as the concept of "maximum drawdown" is not meaningful.
However, if we can't say what risk is ahead of us, we can judge and assess properly whatever risk is behind us, and that is, 12 days ago, 77% is on our side. And now, at 9000, about 97% (with all the premises and assumptions made above).
As capital will increase, the risk of blowing out will keep decreasing, but, until the end, I will never be safe from it, unless of course, I decide that if I get too unlucky, I quit trading. But, even if I do that after being so unlucky as to start on the worst day in a million, I will still not know if my systems have failed or if they've been extremely unlucky.
So, we're basically proceeding by guesstimates, because even finding a "profitable" system is a rule-of-thumb process, as you could never exclude for sure that your system is wrong and has just been unlucky and viceversa.
Having said this, in practice, since I can't talk like I'm writing an essay all the time, how did I benefit from this probability reading?
According to my previous faulty thinking, I would not have started trading because I would have seen a 10k drawdown as the maximum drawdown, and said "hey, I will only be safe with 12k". Instead, I started, and this saved me time. But increased risk, so this is not that much of an advantage except for the fact that it gave me confidence as to the risks I would be running, and confidence is part of doing the job right.
The other benefit from probability thinking, and the most important one, is that, I used tables like the above to detect the optimal portfolio. And, unlike I did in the past, this time, I did not look for the portfolio of systems with the
instance of the lowest drawdown but for the system with the
least frequent and lowest drawdown. And this final point is the
most important point of the whole post and of the whole probability thinking benefits.
You see, the most important is this: is it better to select a portfolio that, by starting trading on a given day, has 23% of chances to make you lose from 3k to 18k (thereby blowing out my account), or a portfolio that has 46% chance of losing from 3k to 10k (thereby, just the same, blowing out my account)? The best portfolio is clearly the first one, which however, by the common "maximum drawdown" thinking, would be the worst one. Yet, my reading up on probability made me understand, that it was better to enable 12 systems and opt for the first portfolio, than for the second, apparently "safer" one, which only trades 4 systems. Also that wikinvest article would have made you choose the safer one.
Yet the first one, not only propels itself like a rocket faster into the sky, but it also makes more money - obviously the two things are connected. So I choose a portfolio that makes more money and is less likely to blow out my account, given that at any rate, I could not lose more than 2000, and before having 10k, I would have had to wait over a year. So, what I am saying is that, for a given level of capital, you can choose an optimal portfolio according to probability think that is totally different, and much better, from the one you would have chosen based on "maximum drawdown" thinking.
Rather than a comparison between "probability" on the one side and "maximum drawdown", since I want to simplify things and be logical, and use the most common terms, I would define it a comparison between "maximum drawdown" and "frequency of maximum drawdowns" as methods to select a portfolio of trading systems. And I would conclude that the second method is much better, since "maximum drawdown" does not exist to begin with. Ok, and with this perfect summary, I am done.
[...]
Not done yet. This whole post proposed a comparison between two methods for selecting portfolios of trading systems: one which considers the
extent of the biggest fall - max drawdown, which doesn't mean anything, because the sample is limited, and in reality the biggest fall doesn't exist - and the other which considers the
frequency of all the falls. This comparison explains how it is preferable to consider all the falls rather than the biggest fall, by making the following points:
1) you cannot estimate the biggest fall ("maximum drawdown"), which is potentially infinite, but can only estimate the probability of different falls.
2) since you cannot estimate the biggest fall, no capital is ever enough for you to invest safely
3) since, no matter how much money you have, you won't have enough to invest safely, you can only decide how much to risk based on the probabilities you know
4) this equates two people, one with 1 million, but willing to risk 2000, and one with 2000 and willing to risk 2000.
5) if you have a capital of 4000 (that stops trading with a loss of 2000), the best way to select your portfolio is therefore not the portfolio that has the lowest maximum drawdown (which doesn't exist), but the one that has the least probability of making you lose that 2000 dollars, therefore the portfolio with the least frequency of drawdowns above 2000 dollars.
There's much more to selecting a portfolio of trading systems, but so far I can only say this much, which is already a lot. I will need a lot more math to solve other questions.
What amazes me is that I know a few people with a scientific background who didn't even get this far and keep applying that meaningless application of maximum drawdown (which I explained above), and they've gotten me to do the same until recently.
So I guess it is not just a question of how much math you know, but also of how you use it.
[...]
Let's take it even further, in clarifying this whole thing.
In my choice of the systems to be included in the portfolio I had these requirements:
1) my capital was 4000
2) I wanted to make at least 1000 per month
Given these requirements, I had to look for the portfolio with the lowest probability of blowing out. And I found what I found (the 12 systems), but let's review the logical steps I followed.
The resampling came afterwards (I had been trading for 2 days already). I started off by using the relativized back-tested trades to screen for the optimal portfolio.
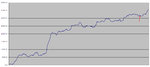
The first choice was to trade just the one system that worked the best (great sharpe ratio, low margin, and low absolute drawdown). Its table looked like this:
This is ok, but it did not make the money I wanted to make, but only 500 per month on average, and I would have spent months without any action. Not good enough.
So I kept adding many other very good systems, and combinations thereof, and realized that they did not make enough money AND they had a higher probability of blowing out than the whole 12 of them put together:
So the trick, and it's really counter-intuitive, is that the (deceitful concept of) "maximum drawdown" increases as the number of systems traded increases, but the probability of blowing out actually has a tendency to decrease.
This, I suppose, happens because the rate at which the drawdown increases is not as strong as the rate at which profit increases, so yes, there will be bigger falls if you trade 12 systems than if you trade 6, as a tendency, but there will be many more rises, too, and, given the profitability of the systems, the rises will more than compensate the falls. So, when the bigger falls happens, you're much higher up in the equity line, and the bigger drawdown affects you less.
Ultimately, by trading more (profitable) systems, you tend to, at once, make more money and risk less. And by increasing the absolute maximum drawdown of your (profitable) portfolio, you're actually decreasing your probability of blowing out the account. It is very counter-intuitive but it tends to happen.
Because there will be situations where the greater amount of losses from the great amount of systems will combine to produce a greater amount of drawdown, but, given that the systems are profitable, these greater losses will be
more than compensated by the more frequent situations where the greater amount of wins from the greater amount of systems will combine to produce greater amount of "draw-ups" (rises in the equity curve), which will more than compensate the increased drawdown, which is only increased in absolute terms and not in % terms - and I think but it's only a guesstimate that this is caused by the positive effect of diversification (rather than by the law of large numbers).