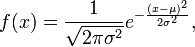Yamato
Legendary member
- Messages
- 9,840
- Likes
- 246
The meaning of "risk"
This is fascinating. It's like mental weight-lifting. You never want to do it, but if you do it, you start seeing improvements pretty quickly. In my case I wish it were like swimming, which i find enjoyable while useful to get into shape. Unfortunately, reading textbooks and formulas is not exactly as enjoyable and natural as swimming is for me.
So. I've been reading, Bernstein in particular. And the ideas I received started growing in my mind.
This time I'd like to talk about "risk".
When we talk about risk in finance, it means or at least it sounds like "risk of losing money". The way it always sounded to me was the "risk of losing money permanently", in other words "bankruptcy", "blowing out your account", "losing all your money".
Then I was reading Bernstein and his constant talk about stocks' superior return and for a second I said to myself: wait a minute, is "risk" just a synonym for "drawdown", "temporary loss of money"?
Yeah, because he kept saying (like many others do) that taking risks gets rewarded by a bigger return, and kept showing examples of how, overall, by investing in stocks you make more money, so one wonders if "risk" just means "drawdown".
But then I read about the "uncle fred" example, and it ends like this:
CHAPTER ONE
So, he's finally confirming to me, after going through dozens of pages, that "risk" isn't merely the "gut wrenching" part of variability in returns, but there is also a part where you "risk" not making as much money with bonds, and there is also a part where you "risk" losing everything, which is bigger with stocks than with bonds.
But then, if it is indeed like this, and there are no doubts that i am getting it right, he makes a mistake soon thereafter in chapter one:
CHAPTER ONE
where he says:
He should have said: "in the long run you are PROBABLY compensated for bearing risk EXCEPT when you lose everything".
The tradeoff between risk and return is of a nature whereby if you invest in one individual bond, you're actually risking more than if you're investing in 100 stocks.
First, because you will make less money, so we can say "you risk a lower return", given that the term "risk" is so abused anyway, let's mention all its aspects and implications.
Second, because the government (Argentina, for example) could go bankrupt and not pay you back, whereas a basket of stocks from all over the world would be safer.
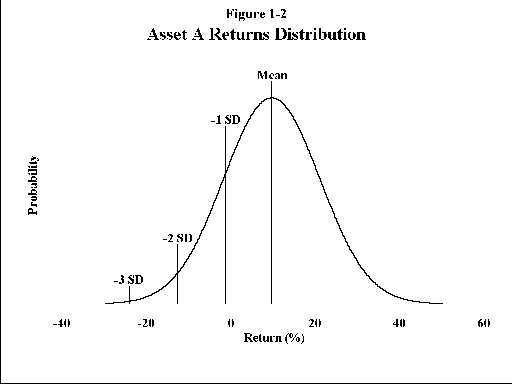
So this is where I guess the "efficient frontier" (famous chart) comes into play. There's ways and ways to balance risk with return. You could invest in one bond and risk more than by investing in 10 stocks.
But yes, all other things being equal, a balanced portfolio of stocks yields more and implies a higher risk than a balanced portfolio of bonds.
And also, the longer term is your investment, just like tossing the coin, the more likely you are to benefit from stocks than from bonds.
So what these guys are saying, given the "uncle fred" example and all that, is that with stocks you are going to have a majority of profitable years, a minority of unprofitable years, and also the chance that during your lifetime you will be so unlucky as to lose... even everything. With bonds, you are going to have lower returns and pretty much the same exact thing, except the risk of losing everything is much lower, and the risk of not making any money is also lower.
So, all in all, we can say: in the long run there is a tendency to be compensated for bearing intelligent risk.
It is pretty far from what he said. But I'll keep reading his stuff nonetheless, and i owe him for his clarity.
But you see, we need to define once and for all this "risk" term, because it's still pissing me off.
How can one say "you're compensated for bearing risk in the long run"? That is contrary to any common sense interpretation of the word "risk". They don't really mean "take risks and you'll make money". What they mean is "if you diversify your portfolio and keep it for the long term, you will reduce your risks of losing everything so much and increase your probability of making much more money so much, that it will make sense to invest in stocks (or in a mix of stocks and bonds) rather than just bonds.
I am still not good enough to sum it up in a formula, but:
1) their concept of more "risk" does mean a higher risk of losing everything
2) but also their concept of more "risk" (despite the apparent contradiction) implies a higher probability of making money (with stocks)
So we're increasing the "risk" of a bad thing happening at a lower rate and at a lower speed than we're increasing the probability of a good thing happening.
So they should not make it sound like we're just increasing risk (of a bad thing). They should also stress out, each time, that we're increasing the probability of a good thing.
The problem with all their simplifications (bernstein's and markowitz's) is that they do not... not they... but the English and financial terms... they do not convey that we're not just increasing risk but we're also increasing the probability of a good outcome. So with this concept of doing something "risky" they make it impossible for any ignorant and superficial person like me to really grasp what the **** they might be talking about.
It's like the famous "no pain no gain"... I mean all this crap like "risk is rewarded"... this all sounds like "run to your death" and so obviously it doesn't make any sense to anyone who's unable to go beyond the appearance of words.
If you do so, you realize, you finally realize that what they are implying BUT NOT saying (the mother ****ers) is, as you accept a (slightly) higher risk of losing everything, if you are doing everything right and diversifying and holding for the long term, you will highly increase your probability of making a lot more money.
Goddamn mother ****ers.
Probably, because of the stupid journalists (because they're the ones spreading the superficial and dangerous bits of knowledge, here and there) the average moron will go to the bank and buy just argentinian bonds and feel secure. Then the other moron will also misinterpret it and go and buy a couple of stocks.
Anyway.
Another major point that Bernstein makes (but not in chapter one - nope he beats about the bush for several chapters) is that stock picking is useless. All that matters is a good balance of asset classes. The same thing more or less that markowitz seems to say.
Now, how does all this apply to my 120 systems?
Very much so, but let me think about it for a while.
Well, some brainstorming.
First of all, my trading systems would seem like a bunch of stocks, and they'll be even less correlated than stocks are. But then they might not be as good as all those asset classes that Bernstein cites (but in the other book, "The Investor's Manifesto"):
Second of all, my systems are good enough to not need to be traded along with bonds in a diversified portfolio. By all means they will make money every single year.
Yes, as the "risk" term implies, there's a slightly higher risk that my systems will lose everything than if you simply bought a basket of bonds.
I haven't gotten so far as to read their recipe for diversification and limiting risk. But, once I do, I will have to learn their approach (and formulas) regarding how to avoid the pitfall of expecting a portfolio that was balanced in the past to necessarily work in the future. I will need to adapt such an approach to my systems. Whatever I do, this reasoning will help me, provided I get done with it. I can't leave this task unfinished or it will just make me more insecure with regard to math ("i tried again and failed again").
This is fascinating. It's like mental weight-lifting. You never want to do it, but if you do it, you start seeing improvements pretty quickly. In my case I wish it were like swimming, which i find enjoyable while useful to get into shape. Unfortunately, reading textbooks and formulas is not exactly as enjoyable and natural as swimming is for me.
So. I've been reading, Bernstein in particular. And the ideas I received started growing in my mind.
This time I'd like to talk about "risk".
When we talk about risk in finance, it means or at least it sounds like "risk of losing money". The way it always sounded to me was the "risk of losing money permanently", in other words "bankruptcy", "blowing out your account", "losing all your money".
Then I was reading Bernstein and his constant talk about stocks' superior return and for a second I said to myself: wait a minute, is "risk" just a synonym for "drawdown", "temporary loss of money"?
Yeah, because he kept saying (like many others do) that taking risks gets rewarded by a bigger return, and kept showing examples of how, overall, by investing in stocks you make more money, so one wonders if "risk" just means "drawdown".
But then I read about the "uncle fred" example, and it ends like this:
CHAPTER ONE
Uncle Fred’s coin toss may seem a most bizarre scenario, and yet it is nearly identical to the choice faced by most investors between the "safety" of money market accounts/treasury bills and the "gamble" of common stocks. The second option offers a near certainty of a superior result, yet comes at a price: the small possibility of an inferior result and, more importantly, that gut wrenching coin toss session with Uncle Fred each year. And yet, it is the 3% certificate of deposit option which is the most truly frightening—you will almost certainly live your golden years in poverty.
So, he's finally confirming to me, after going through dozens of pages, that "risk" isn't merely the "gut wrenching" part of variability in returns, but there is also a part where you "risk" not making as much money with bonds, and there is also a part where you "risk" losing everything, which is bigger with stocks than with bonds.
But then, if it is indeed like this, and there are no doubts that i am getting it right, he makes a mistake soon thereafter in chapter one:
CHAPTER ONE
where he says:
You have just been introduced to one of the fundamental laws of investing: in the long run you are compensated for bearing risk.
He should have said: "in the long run you are PROBABLY compensated for bearing risk EXCEPT when you lose everything".
The tradeoff between risk and return is of a nature whereby if you invest in one individual bond, you're actually risking more than if you're investing in 100 stocks.
First, because you will make less money, so we can say "you risk a lower return", given that the term "risk" is so abused anyway, let's mention all its aspects and implications.
Second, because the government (Argentina, for example) could go bankrupt and not pay you back, whereas a basket of stocks from all over the world would be safer.
So this is where I guess the "efficient frontier" (famous chart) comes into play. There's ways and ways to balance risk with return. You could invest in one bond and risk more than by investing in 10 stocks.
But yes, all other things being equal, a balanced portfolio of stocks yields more and implies a higher risk than a balanced portfolio of bonds.
And also, the longer term is your investment, just like tossing the coin, the more likely you are to benefit from stocks than from bonds.
So what these guys are saying, given the "uncle fred" example and all that, is that with stocks you are going to have a majority of profitable years, a minority of unprofitable years, and also the chance that during your lifetime you will be so unlucky as to lose... even everything. With bonds, you are going to have lower returns and pretty much the same exact thing, except the risk of losing everything is much lower, and the risk of not making any money is also lower.
So, all in all, we can say: in the long run there is a tendency to be compensated for bearing intelligent risk.
It is pretty far from what he said. But I'll keep reading his stuff nonetheless, and i owe him for his clarity.
But you see, we need to define once and for all this "risk" term, because it's still pissing me off.
How can one say "you're compensated for bearing risk in the long run"? That is contrary to any common sense interpretation of the word "risk". They don't really mean "take risks and you'll make money". What they mean is "if you diversify your portfolio and keep it for the long term, you will reduce your risks of losing everything so much and increase your probability of making much more money so much, that it will make sense to invest in stocks (or in a mix of stocks and bonds) rather than just bonds.
I am still not good enough to sum it up in a formula, but:
1) their concept of more "risk" does mean a higher risk of losing everything
2) but also their concept of more "risk" (despite the apparent contradiction) implies a higher probability of making money (with stocks)
So we're increasing the "risk" of a bad thing happening at a lower rate and at a lower speed than we're increasing the probability of a good thing happening.
So they should not make it sound like we're just increasing risk (of a bad thing). They should also stress out, each time, that we're increasing the probability of a good thing.
The problem with all their simplifications (bernstein's and markowitz's) is that they do not... not they... but the English and financial terms... they do not convey that we're not just increasing risk but we're also increasing the probability of a good outcome. So with this concept of doing something "risky" they make it impossible for any ignorant and superficial person like me to really grasp what the **** they might be talking about.
It's like the famous "no pain no gain"... I mean all this crap like "risk is rewarded"... this all sounds like "run to your death" and so obviously it doesn't make any sense to anyone who's unable to go beyond the appearance of words.
If you do so, you realize, you finally realize that what they are implying BUT NOT saying (the mother ****ers) is, as you accept a (slightly) higher risk of losing everything, if you are doing everything right and diversifying and holding for the long term, you will highly increase your probability of making a lot more money.
Goddamn mother ****ers.
Probably, because of the stupid journalists (because they're the ones spreading the superficial and dangerous bits of knowledge, here and there) the average moron will go to the bank and buy just argentinian bonds and feel secure. Then the other moron will also misinterpret it and go and buy a couple of stocks.
Anyway.
Another major point that Bernstein makes (but not in chapter one - nope he beats about the bush for several chapters) is that stock picking is useless. All that matters is a good balance of asset classes. The same thing more or less that markowitz seems to say.
Now, how does all this apply to my 120 systems?
Very much so, but let me think about it for a while.
Well, some brainstorming.
First of all, my trading systems would seem like a bunch of stocks, and they'll be even less correlated than stocks are. But then they might not be as good as all those asset classes that Bernstein cites (but in the other book, "The Investor's Manifesto"):
The first column simply lists the asset classes we are
examining; the first 12 of them are the major foreign and
domestic equity (that is, stock) classes, separated by three
different criteria: location (United States, foreign developed
nations, and foreign emerging - market nations), company
size (large versus small), and whether the companies
are of the “ value ” or “ market ” type.
Second of all, my systems are good enough to not need to be traded along with bonds in a diversified portfolio. By all means they will make money every single year.
Yes, as the "risk" term implies, there's a slightly higher risk that my systems will lose everything than if you simply bought a basket of bonds.
I haven't gotten so far as to read their recipe for diversification and limiting risk. But, once I do, I will have to learn their approach (and formulas) regarding how to avoid the pitfall of expecting a portfolio that was balanced in the past to necessarily work in the future. I will need to adapt such an approach to my systems. Whatever I do, this reasoning will help me, provided I get done with it. I can't leave this task unfinished or it will just make me more insecure with regard to math ("i tried again and failed again").
Last edited: