Skill's weekend teaser
- Thread starter Skill Leverage
- Start date
- Watchers 19
dick_dastardly
Established member
- Messages
- 843
- Likes
- 152
I'm not going to read through 50 pages so I'm sorry if I'm repeating what's already been said.
The moment the plane wheels turn the conveyor responds by speeding up, which then causes the plane wheels to rotate faster and then the conveyor responds by speeding up again and so on and so on ( or have I misread the question ).
To me that's a positive feed back loop ( unstable system ) which will ramp up to infinity the moment the plane wheels start to rotate.
The components of the conveyor and/or the plane undercarriage will centrifuge and disintigrate or seize up due to over heating.
If the conveyor and the plane wheels are theoretically perfect then it's a paradox surely ???
thick_dastardly
The moment the plane wheels turn the conveyor responds by speeding up, which then causes the plane wheels to rotate faster and then the conveyor responds by speeding up again and so on and so on ( or have I misread the question ).
To me that's a positive feed back loop ( unstable system ) which will ramp up to infinity the moment the plane wheels start to rotate.
The components of the conveyor and/or the plane undercarriage will centrifuge and disintigrate or seize up due to over heating.
If the conveyor and the plane wheels are theoretically perfect then it's a paradox surely ???
thick_dastardly
Skill Leverage
Experienced member
- Messages
- 1,316
- Likes
- 186
lol thick_dastardly, very good...
You are no such thing however, because you are correct. The original post was worded poorly and this was pointed out somewhere in the wreckage of this thread. Better wording would be to say 'the same speed as the plane, but in the opposite direction'.
TBH I didn't pay that much attention to that aspect of the wording when I drew it from my notes, purely because I knew that the speed is irrelevant in the context of the problem. You are right however, and I would have edited the OP if I had time in between bouts of crying into my pillow.
SL
You are no such thing however, because you are correct. The original post was worded poorly and this was pointed out somewhere in the wreckage of this thread. Better wording would be to say 'the same speed as the plane, but in the opposite direction'.
TBH I didn't pay that much attention to that aspect of the wording when I drew it from my notes, purely because I knew that the speed is irrelevant in the context of the problem. You are right however, and I would have edited the OP if I had time in between bouts of crying into my pillow.
SL
new_trader
Legendary member
- Messages
- 6,770
- Likes
- 1,656
Give up trying to explain why the plane will take off because for some people it will never land!
TheBramble
Legendary member
- Messages
- 8,394
- Likes
- 1,170
We have agreed the plane must reach take off speed to take off.
We have agreed the plane can only reach take off speed by moving itself through the air.
We agree that for it to have brought itself from a standing start to take off speed it much have travelled some distance from its starting point – we called that X.
We accept from you post #1 that the conveyor belt and the wheels of the plane are (in some way that is not defined, but a given) are arranged such that the conveyor moves backward precisely and exactly in unison with the wheels rotating forward.
So for any forward movement of the plane, X for instance, the conveyor will have moved backward X in that same period of time.
The net movement of the plane under the circumstances you lay down, is zero. It’s at its starting point. It can never be anywhere other than at its starting point under the conditions you specify.
It’s not aerodynamics, or Newtons 2nd Law or Physics or anything other than simple bloody arithmetic. +X-X = 0
However unlikely or impossible this is in reality – that’s how Skills posed the question.
For those that start blending in real-life effects and issues such as friction, ice, thrust against the air etc. slap your self (or Skills) in the head and look at post #1 again, and again until you realise this is a theoretical situation set for us by skills and no element of reality should be allowed to cloud your judgement.
A jet blowing full power at the end of a runway will NOT take off unless it is able to translate that thrust into forward motion. However that forward motion is impeded - we don’t know how Skills managed it nor do we care – is completely irrelevant. He tells us it is by the simple expedient of ensuring the rotation of the wheels is exactly matched my the opposite motion of the conveyor. We accept that.
The plane does not take off.
We have agreed the plane can only reach take off speed by moving itself through the air.
We agree that for it to have brought itself from a standing start to take off speed it much have travelled some distance from its starting point – we called that X.
We accept from you post #1 that the conveyor belt and the wheels of the plane are (in some way that is not defined, but a given) are arranged such that the conveyor moves backward precisely and exactly in unison with the wheels rotating forward.
So for any forward movement of the plane, X for instance, the conveyor will have moved backward X in that same period of time.
The net movement of the plane under the circumstances you lay down, is zero. It’s at its starting point. It can never be anywhere other than at its starting point under the conditions you specify.
It’s not aerodynamics, or Newtons 2nd Law or Physics or anything other than simple bloody arithmetic. +X-X = 0
However unlikely or impossible this is in reality – that’s how Skills posed the question.
For those that start blending in real-life effects and issues such as friction, ice, thrust against the air etc. slap your self (or Skills) in the head and look at post #1 again, and again until you realise this is a theoretical situation set for us by skills and no element of reality should be allowed to cloud your judgement.
A jet blowing full power at the end of a runway will NOT take off unless it is able to translate that thrust into forward motion. However that forward motion is impeded - we don’t know how Skills managed it nor do we care – is completely irrelevant. He tells us it is by the simple expedient of ensuring the rotation of the wheels is exactly matched my the opposite motion of the conveyor. We accept that.
The plane does not take off.
KillPhil08
Experienced member
- Messages
- 1,860
- Likes
- 381
YouTube - Mythbusters - Plane on conveyor belt the practice!
please note the guy at 3:40 shouting.
I WON!!
please note the guy at 3:40 shouting.
I WON!!
KillPhil08
Experienced member
- Messages
- 1,860
- Likes
- 381
It's not looking good, Bramble
So my Physics is rusty to say the least, but I do believe herein lies a proof of the solution.
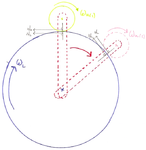
The conveyor belt is analagous to the lower Roller, the wheels of the plane the upper. The Red bar joins the centre of rotation of the two rollers, and is free istelf to rotate about the centre of the lower roller.
The angular velocity of the lower roller is set such that the velocity of a point tangental to the rollers is equal.
The problem is then to show that by applying a torque about the Red bar, the angular velocity of the bar is non-zero, while the condition V(u) = V(l).
As the angular frequency of the upper and lower rollers are a function of one another, you can express W(l) in terms of W(u) - then show that the angular acceleration about the centre by the red bar (and therefore the centre of rotation of the upper roller) is NOT a function of how fast the either the upper or lower roller is rotating (i.e., the terms V(u) and V(l) , or W(l) and W(u), will "drop out" of the expression).
It should be clear that applying a torque about the red bar has exactly the same effect as sticking a jet engine on the axle of the upper roller; so while the rollers cannot move relative to one another (i.e. if you were to set markers along the circumference, they would still line up), but the upper roller CAN move translationally with respect to the centre of rotation of the lower roller.
So my Physics is rusty to say the least, but I do believe herein lies a proof of the solution.
The conveyor belt is analagous to the lower Roller, the wheels of the plane the upper. The Red bar joins the centre of rotation of the two rollers, and is free istelf to rotate about the centre of the lower roller.
The angular velocity of the lower roller is set such that the velocity of a point tangental to the rollers is equal.
The problem is then to show that by applying a torque about the Red bar, the angular velocity of the bar is non-zero, while the condition V(u) = V(l).
As the angular frequency of the upper and lower rollers are a function of one another, you can express W(l) in terms of W(u) - then show that the angular acceleration about the centre by the red bar (and therefore the centre of rotation of the upper roller) is NOT a function of how fast the either the upper or lower roller is rotating (i.e., the terms V(u) and V(l) , or W(l) and W(u), will "drop out" of the expression).
It should be clear that applying a torque about the red bar has exactly the same effect as sticking a jet engine on the axle of the upper roller; so while the rollers cannot move relative to one another (i.e. if you were to set markers along the circumference, they would still line up), but the upper roller CAN move translationally with respect to the centre of rotation of the lower roller.
Attachments
new_trader
Legendary member
- Messages
- 6,770
- Likes
- 1,656
Where do babies come from?
Where do babies come from?
Thats something to do with purple rods.
TheBramble
Legendary member
- Messages
- 8,394
- Likes
- 1,170
We don't need to resort to analgous constructs.
edit: maybe this might help. Post #1, Skills says the wheels' rotation will be exactly offset by the opposite motion of the conveyor. The wheels WILL turn, but the axel upon which the wheels are turning will not move relative to any fixed reference point.
edit: maybe this might help. Post #1, Skills says the wheels' rotation will be exactly offset by the opposite motion of the conveyor. The wheels WILL turn, but the axel upon which the wheels are turning will not move relative to any fixed reference point.
Last edited:
Explain to me why the two scenarios must behave differently.
tightstops
Active member
- Messages
- 245
- Likes
- 19
anyone remember what page the explanation was on. dont fancy reading 53 pages. thanks
trendie
Legendary member
- Messages
- 6,875
- Likes
- 1,433
use the "Search this thread" facility to read onyl Skill Leverages posts.
somewhere in there is the answer. and much less than 53 pages.
watch as he becomes increasingly apoplectic in his replies and his growing rage at the posters.
new_trader
Legendary member
- Messages
- 6,770
- Likes
- 1,656
I have a brilliant idea! Perhaps 'The Bramble' should re-write the question so that it fits your solution.
new_trader
Legendary member
- Messages
- 6,770
- Likes
- 1,656
If I had the ability to integrate the solution over all rollers, I would prove it (but, of coure, I don't).
Despite what the diagram looks like, the conyeyor belt can be considered as the surface of a rotating cylinder, and the thrust of the engines can be considered as a tourque about a point.
Despite what the diagram looks like, the conyeyor belt can be considered as the surface of a rotating cylinder, and the thrust of the engines can be considered as a tourque about a point.
megamuel
Experienced member
- Messages
- 1,098
- Likes
- 164
TheBramble is gonna get so many reputation points when he comes round cos its gonna take some serious balls! Brambles do you still think that the jet engines can't make the plane fly if its on a conveyor belt runway? What would it take for you to change your mind? If I can get Stephen Hawking to answer by emailing his website, will you believe it then? Or would the only proof be seeing it actually happen? I don't think I can arrange that, although my brother does work for Hawker Beechcraft! I must say, I do admire your perserverence!
Sam.
Sam.
TheBramble
Legendary member
- Messages
- 8,394
- Likes
- 1,170
Well, that's the thing GJ. I wouldn't want to standing in front of it because if I was, I'd be stading on the conveyor which is moving under it. I'm sure there's more than intelligence the issue here as I know all (well most) of you guys can do basic arithmetic.
+x-X = 0.
The plane does not take off.
+x-X = 0.
The plane does not take off.
Similar threads
- Article
- Article
